Hamilton Lecture explores beauty of hyperbolic geometry

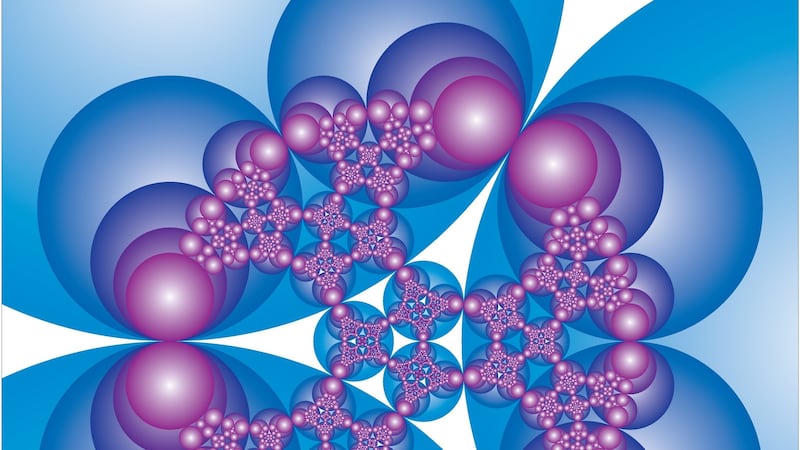
Mathematicians often comment on the beauty of their chosen discipline. For the non-mathematicians among us, that can be hard to visualise. But in Prof Caroline Series’s field of hyperbolic geometry, the beauty is instantly rendered in the dazzling patterns that it creates.

On Friday, October 15th, Series will give a glimpse into that beauty when she delivers the 2021 Hamilton Lecture online. But first things first – what is hyperbolic geometry?

"It is geometry on a certain kind of curved surface," explains Series, who is professor emeritus of mathematics at Warwick University. "At school we learn about ordinary, Euclidean geometry, where we imagine shapes, such as triangles, on a flat surface. Now imagine spherical geometry, on a sphere, which we might use for navigation around the globe. Then, in hyperbolic geometry, you might have a surface that is curved in a different way, shaped like a saddle or a trombone or bugle. In hyperbolic geometry, we have formulas for working out what happens with shapes on this kind of surface."

As hyperbolic shapes grow and take up space, they can twist and turn in different ways, explains Series, and they may become crinkled in order to ‘fit’ at the edges. “An example in nature is the leaves of curly kale,” she says. “As the leaves get larger, you see these frills and folds forming at the edges.”

Fifth Mary Mulvihill Award offering €2,000 for best entry on theme of water

---

Green leaves and tough grasses – how plants respond to climate change

---

Trinity College’s ‘wildflower meadow’: What it was trying to achieve

---

Harnessing machine learning to help patients with ALS

---

Hyperbolic goes hyperbolic

The annual Hamilton Lecture celebrates the legacy of Irish mathematician William Rowan Hamilton. While walking along the Royal Canal in October 1843, he inscribed details of a new type of equation called quaternions into the stonework at Broome Bridge in Cabra. Quaternions, which allow computation in higher dimensions, are in the background of hyperbolic geometry, Series notes. This and many other insights in the 19th and 20th centuries advanced the field.

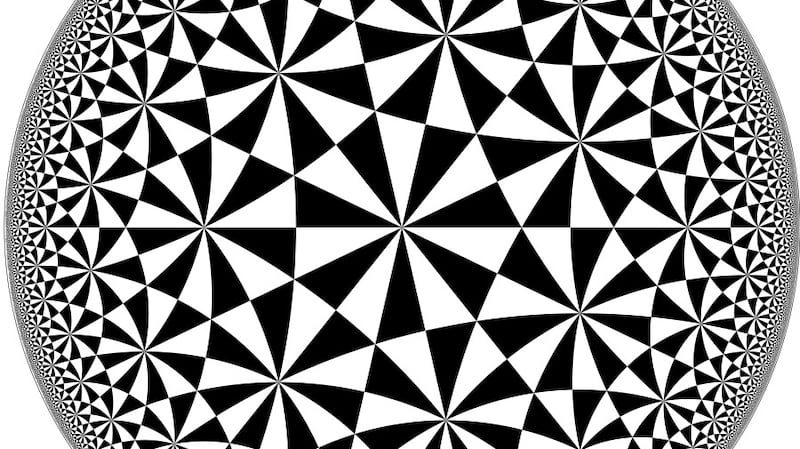
"There was one genius in particular, William Thurston, who had fabulous ideas about three-dimensional shapes and knots," she says. "In the 1970s and 1980s, he discovered that many of these shapes intrinsically carried this kind of hyperbolic geometry. His students went on and developed these ideas, helped by more powerful computers, and hyperbolic geometry became more mainstream."

Computer graphics based on hyperbolic geometry show curving patterns of tiles, drawing the eye to their complex edges. It was one such image that attracted Series to start working in the field. “I was in a colleague’s office and he had one of these images hanging on the wall, and I was fascinated by it,” recalls Series, who later co-authored the book Indra’s Pearls, which explores hyperbolic images. “I have been more or less working on hyperbolic geometry ever since.”

Solving problems

Twisting your head around these complicated, three-dimensional shapes is not easy, but the key to solving the puzzles in hyperbolic geometry is to be creative and break them down, according to Series, who was elected as a fellow of the Royal Society in 2016.

“Mathematics is incredibly powerful,” she says. “The trick is to reduce something that is very complicated down to steps that are simple and routine, so that you have a method of dealing with them.”

Hyperbolic geometry is proving useful in applications such as managing complex hierarchical datasets and even figuring out how our sense of smell gathers information from chemicals in the air, Series adds.

“I am a pure mathematician, I love the way that mathematics is used in so many things but I don’t do that myself,” she says. “But I find it interesting that if you try to represent data that are hierarchical in hyperbolic space, because there is more room there, the data can be represented in a more natural way. A lot of what people do now is trying to find the right geometry to deal with the particular data being represented.”

She also sees the power of maths to understand everyday problems and crises – whether it’s figuring out how to avoid fuel shortages or modelling the spread of Covid-19. “Maths is in everything nowadays,” she says. “And that’s why it is so important to know about this universal language.”

The 2021 Hamilton Lecture 'Glimpses into Hyperbolic Geometry' by Prof Caroline Series, hosted by the Royal Irish Academy in association with Ibec and The Irish Times, takes place online at 7pm on Friday, October 15th. Register for free at ria.ie/events